

It is a beautiful Spring Morning. Everywhere you look, people are happily going to their classes, or coming from their classes. "College is fun!'' calls out one student, and about twenty more yell "Sure is!'' in unison. Someone else calls out, "I love History!" A bunch of other students call "Great subject!'' in response. Swept up in the spirit of things, you call out, "Calculus is wonderful!" "Lies! Lies!'' calls out one familiar voice. You wheel around and directly behind you is a wild-eyed hungry looking stranger.
"Oh, don't be silly,'' you say. "I just learned about trigonometric integration. It wasn't that hard a section, and there isn't a single lie in it.''
He looks up at you and says, "Oh, really? Perhaps you can take a quick true-or-false test, and see how easy the section is." The stranger then whips out a sheet of paper with this on it:
;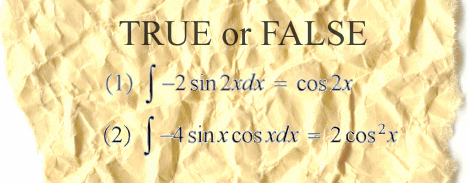
"Both are clearly true!'' he shouts, before you have a chance to think. "AND we know that -2sin(2x) = -2 (2sin xcos x) = -4sin x cos x! Thus cos 2x=2cos2x! Ho ho!''
"Ho ho?'' you ask.
"Ho ho, I say! Ho, ho I mean! Because at x = 0, cos 2x = 1, and 2cos2x=2. Once again, your "Calculus'' gets you into trouble! 2=1! 2=1!" At that, the stranger skips off into the distance.
Consider the stranger's test. Are the answers "true'' to both questions? And if so, then could the stranger be correct? If 1=2, then how can you tell odd numbers from even ones? Would 1 still be the loneliest number? Or is there a possibility that there is an error somewhere in the strangers reasoning? Find the error.
Back to Find The Error!
Go to Doug Shaw's Professorial page
Copyright 2001 by Douglas Shaw